Browse by Topics
- English Worksheets
- Kid's Corner
- Numbers & Operations
- Addition
- Subtraction
- Multiplication
- Division
- Decimals
- Place Value
- Roman Numerals
- Skip Counting
- Odd & Even Numbers
- Patterns
- Cardinal & Ordinal Numbers
- Rounding Numbers
- Estimation of Numbers
- Estimation of Time & Money
- Counting & Cardinality
- Comparing Numbers
- Ordering Numbers
- Fractions
- Prime & Composite Numbers
- Squares & Cubes
- Divisibility Rules
- Factors & Multiples
- Data Handling
- Algebra
- Ratio
- Proportion
- Order of Operations
- Scientific Notation
- Exponents
- Algebraic Expressions
- Evaluating Algebraic Expressions
- Simplifying Algebraic Expressions
- Graphing Lines
- Point Slope Form
- Two Point Form
- Two Intercept Form
- Equations
- Identifying Functions
- Evaluating Functions
- Function Table
- Domain and Range
- Trigonometric Charts
- Quadrants
- Polynomials
- Measurement
- Geometry
- Word Problems
Medians and Centroid of a triangle Worksheets
A Median of a triangle is a line segment from one vertex to the mid point on the opposite side of the triangle. The medians of a triangle are concurrent and they intersect in one common point.
The Centroid of a triangle is the point of intersection of all the three medians of a triangle. The centroid always divides each median into two parts, in the ratio 2:1.
Medians and Centroid of a triangle worksheets free download pdf
-
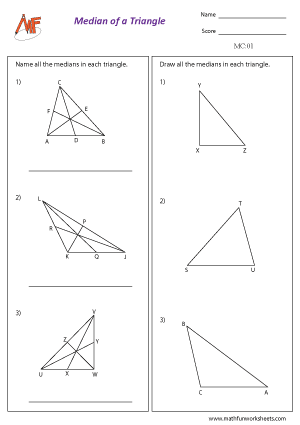
Identify / Draw - Medians of a Triangle
Identify / Draw - Medians of a Triangle
-
Find the Unknown side of a Triangle
Find the Unknown side of a Triangle
-
-
Find the Equation of the Median
Find the Equation of the Median
-
Find the Centroid of the Triangle
Find the Centroid of the Triangle
-
Find the Missing Vertex - From Centroid
Find the Missing Vertex - From Centroid
-
Median and Centroid of a Triangle
Median and Centroid of a Triangle
